Filed Under #teaching
Oct 31, 2023
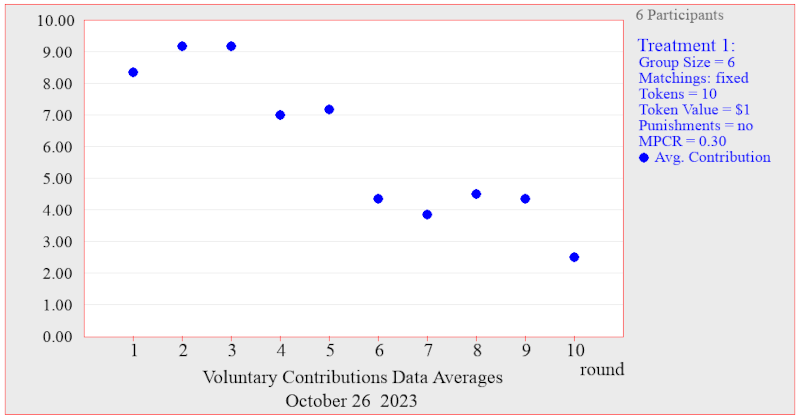Each year since 2017, I’ve been taking one lecture for Ryan’s subject LSM4255 Methods in Mathematical Biology, teaching evolutionary game theory and adaptive dynamics. After the class, we usually have a tutorial where we play the fishing game, a simulation of a fishery that teaches students about the tragedy of the commons. Screenshot from the fishing game, which was sadly not available this year. Unfortunately, the fishing game was unexpectedly unavailable this year, but I was able to find a very nice last-minute alternative. Charles Holt at the University of Virginia has made available the Veconlab software, a collection of...
Web-based public goods game and other experiments for teaching
Each year since 2017, I’ve been taking one lecture for Ryan’s subject LSM4255 Methods in Mathematical Biology, teaching evolutionary game theory and adaptive dynamics. After the class, we usually have a tutorial where we play the fishing game, a simulation of a fishery that teaches students about the tragedy of the commons. Screenshot from the fishing game, which was sadly not available this year. Unfortunately, the fishing game was unexpectedly unavailable this year, but I was able to find a very nice last-minute alternative. Charles Holt at the University of Virginia has made available the Veconlab software, a collection of...
Jul 14, 2023
For 2 weeks, I’ve been teaching at and attending the AMSI Winter School at QUT (Mastodon thread about it here). The winter school is an annual event for postgrads, early-career researchers, and industry professionals, and about 40 students attended this year. Some students from the Winter School. I was one of four presenters on the theme Modelling Our Changing Biosphere. I taught about evolutionary game theory, and we also learnt about modelling fisheries (with Nokuthaba Sibanda from University of Wellington), reinforcement learning (with Carl Boettiger from University of California), model sloppiness (with Matthew Adams from Queensland University of Technology), and...
AMSI Winter School
For 2 weeks, I’ve been teaching at and attending the AMSI Winter School at QUT (Mastodon thread about it here). The winter school is an annual event for postgrads, early-career researchers, and industry professionals, and about 40 students attended this year. Some students from the Winter School. I was one of four presenters on the theme Modelling Our Changing Biosphere. I taught about evolutionary game theory, and we also learnt about modelling fisheries (with Nokuthaba Sibanda from University of Wellington), reinforcement learning (with Carl Boettiger from University of California), model sloppiness (with Matthew Adams from Queensland University of Technology), and...
Nov 29, 2022
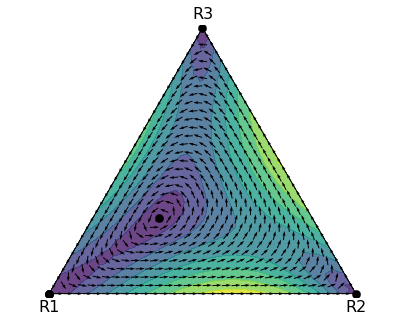Recently, I set a task for a student to use Python to analyse the replicator dynamics of a game with three strategies, including using the Jacobian to determine the stability of the steady state. The purpose of this blog post is to share the solution in case that’s useful to someone. The game For this task, we will consider the replicator dynamics of a 2-player game with 3 strategies. Recall the general equation for the replicator dynamics is \[\begin{equation} \dot{p}_i = p_i (f_i - \bar{f}) \end{equation}\] where \(p_i\) is proportion of \(i\)-strategists in the population, \(f_i\) is fitness effect of...
Example numerical analysis of replicator dynamics with more than 2 strategies
Recently, I set a task for a student to use Python to analyse the replicator dynamics of a game with three strategies, including using the Jacobian to determine the stability of the steady state. The purpose of this blog post is to share the solution in case that’s useful to someone. The game For this task, we will consider the replicator dynamics of a 2-player game with 3 strategies. Recall the general equation for the replicator dynamics is \[\begin{equation} \dot{p}_i = p_i (f_i - \bar{f}) \end{equation}\] where \(p_i\) is proportion of \(i\)-strategists in the population, \(f_i\) is fitness effect of...
Aug 1, 2022
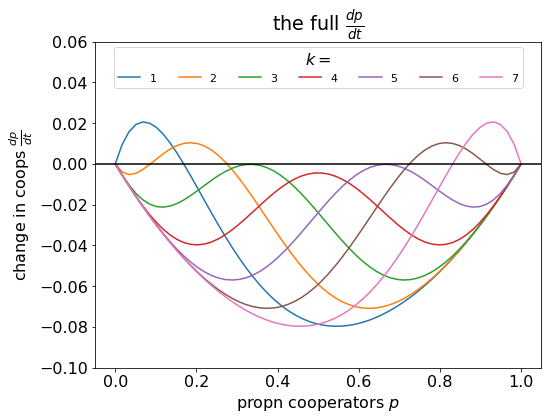Last month, I chose a paper by Kris De Jaegher in Scientific Reports for our weekly lab-meeting discussion. We used the paper to teach ourselves about threshold games, and the purpose of this blog post is to summarise the things we learnt. Replicator dynamics revision De Jaegher used the replicator dynamics approach. Replicator dynamics assumes a well-mixed, infinitely large population of players that reproduces asexually, where the number of offspring they produce depends upon the payoff they receive from a game-theoretic ‘game’, as illustrated below:– Replicator dynamics animation, created by HowieKor (Creative Commons) We start with a population of \(n\)...
Lab meeting about threshold games
Last month, I chose a paper by Kris De Jaegher in Scientific Reports for our weekly lab-meeting discussion. We used the paper to teach ourselves about threshold games, and the purpose of this blog post is to summarise the things we learnt. Replicator dynamics revision De Jaegher used the replicator dynamics approach. Replicator dynamics assumes a well-mixed, infinitely large population of players that reproduces asexually, where the number of offspring they produce depends upon the payoff they receive from a game-theoretic ‘game’, as illustrated below:– Replicator dynamics animation, created by HowieKor (Creative Commons) We start with a population of \(n\)...
Nov 7, 2017
These are the lecture slides for LSM4255 Game Theory and Adaptive Dynamics.
This is the short version with the point-by-point transitions removed.
Downloads:
2023: game_theory_ad2_upload.pdf
2017: game_theory_ad_upload.pdf
Preview of lecture notes.
Lecture notes for LSM4255 Game Theory and Adaptive Dynamics
These are the lecture slides for LSM4255 Game Theory and Adaptive Dynamics.
This is the short version with the point-by-point transitions removed.
Downloads:
2023: game_theory_ad2_upload.pdf
2017: game_theory_ad_upload.pdf
Preview of lecture notes.